Three Phase Diagram Geotechnical Engineering
Soil Phase Relationships
Courses > Soil Mechanics > Physical Properties of Soil > Soil Phase Relationships
 Introduction
Introduction
Soil mass is generally a three-phase system. It consists of solid particles, liquid, and gas. For all practical purposes, the liquid may be considered to be water (although in some cases, the water may contain some dissolved salts) and the gas as air.The phase system may be expressed in SI units either in terms of mass-volume or weight-volume relationships. The interrelationships of the different phases are important since they help to define the condition or the physical make-up of the soil.
 Concepts and Formulas
Concepts and Formulas
Phase relationship diagram:
In a mass of soil, there are three physical components: solid, water, and air. A phase relationship diagram is normally used to represent the relationship as follows:
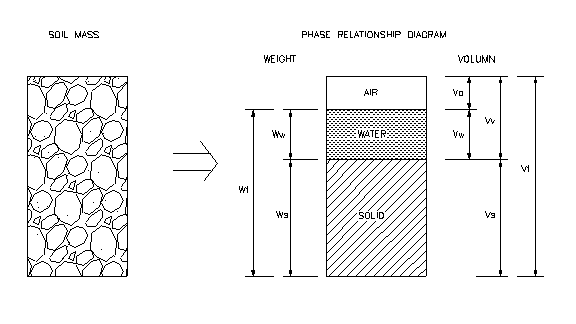
Definitions:
Volume: (ft3, m3)
-
Vt: Total volume
-
Vs: Volume of solid
-
Vv: Volume of void
-
Vw: volume of water
-
Va: Volume of air
Weights: (lbs, kg, kN)
-
Wt: total weight
-
Ws: weight of solid
-
Ww: weight of water
-
Weight of air = 0
Phase Relationships:
Volume-volume relationship:
-
Void ratio (no unit):
-
Porosity (no unit):
-
Degree of saturation (%):
-
air content:
Weight-weight relationship:
- Water (Moisture) content (%):
 Moisture content at fully saturation is not 100%!
Moisture content at fully saturation is not 100%!
100% moisture content means the weight is equally divided into water and solid or in other words the weight of soil particles is equal to the weight of water.
See: Solution of The Value of The Moisture When Fully Saturated
Weight-Volume relationship:
(Unit weight or density, lbs/ft3, g/cm3, kN/m3)
-
Moisture (total) unit weight:
-
Dry unit weight:
-
Solid unit weight:
-
Saturated unit weight (when soil is completely saturated, S = 100%,Va=0):
-
Submerged (buoyant) unit weight (when soil is below ground water table, S = 100%):
 Following relations are very handy in solving problems:
Following relations are very handy in solving problems:
Unit weight to unit weight relationship
-
Specific gravity:
-
(Unit weight of water = 62.4 lbs/ft3 = 1 g/cm3 = 9.8 kN/m3)
-
Average value of Gs for granular soils is 2.65, while the average value of Gs for cohesive soils is 2.80.
 Watch Videos
Watch Videos
 Solved sample problems
Solved sample problems
- Solution of Unit Weight and Its Conversion from Metric Units to SI and US Units
-
Solution of Soil Compaction Check Via The Voids Ratio
-
Solution of The Value of The Moisture When Fully Saturated
Example 1:Determine unit weights, water content, based on known volume and weight (English units)
Given: (English units)
-
Volume of soil mass: 1 ft3.
-
Weight of soil mass at moist condition: 100 lbs
-
Weight of soil after dried in oven: 80 lbs
Requirements:
Determine moist unit weight of soil, dry unit weight of soil, and water content.
Problem solving technique:
- Moist unit weight gt= Wt / Vt (Wt = 100 lbs, Vt=1 ft3, are given)
- Dry unit weight, gd = Ws / Vt (Weight of solid is weight of soil after dried in oven ,Ws = 80 lbs, Vt=1 ft3, are given)
- Water content, w (%) = Ww/Ws (Ws = 80 lbs , weight of water, Ww not known)
- Find weight of water, from phase relationship diagram, Ww = Wt – Ws.
Solution:
-
Moist (total) unit weight, gt = Wt / Vt = 100/1 = 100 pcf (lbs/ft3)
-
Dry unit weight, gd = Ws / Vt = 80/1= 80 pcf (lbs/ft3).
-
Weight of water = 100-80=20 lbs
-
Water (Moisture) content: w (%) = Ww/Ws ´ 100 (%) = 20/80x100% = 25%

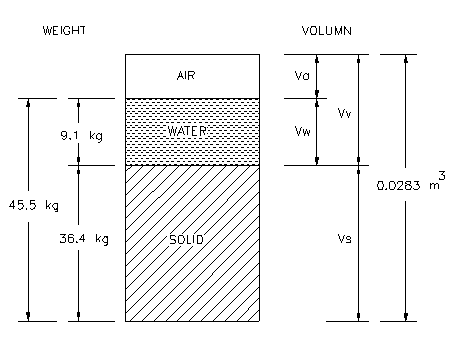
Example 2: Determine unit weights, water content, based on known volume and weight (SI units)
Given: (SI units)
-
Volume of soil mass: 0.0283 m3.
-
Weight of soil mass at moist condition: 45.5 kg
-
Weight of soil after dry in oven: 36.4 kg
Problem solving technique:
- Moist unit weight gt = Wt / Vt (both value are given)
- Dry unit weight, gd = Ws / Vt (both value are given)
- Water content, w (%) = Ww/Ws (Weight of solid is weight of soil after dried in oven is given, weight of water not known)
- Find weight of water, from phase relationship diagram, Ww = Wt – Ws.
Requirements:
Determine moist unit weight of soil, dry unit weight of soil, and water content.
Solution:
-
Moisture (total) unit weight, gt = Wt / Vt = 45.5/0.0283 = 1608 kg/m3 = 1.608 g/cm3
-
Dry unit weight, gd = Ws / Vt = 36.4/0.0283= 1286 kg/m3=1.286 g/cm3
-
Weight of water = 45.5-36.4=9.1 lbs
-
Water (Moisture) content: w (%) = Ww/Ws ´ 100 (%) = 9.1/36.4x100% = 25%
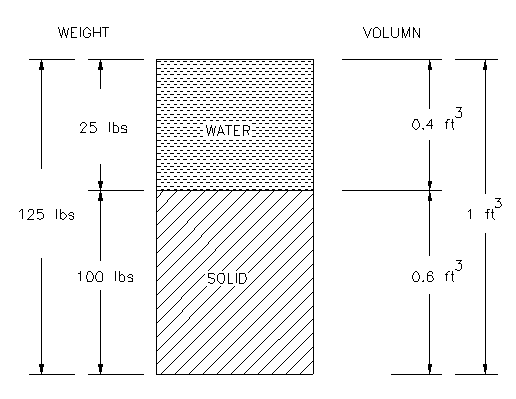
Example 3: Determine void ratio, porosity, and degree of saturation based on known volume, weight, and specific gravity (English units)
Given: (English units)
-
Volume of soil mass: 1 ft3.
-
Weight of soil mass at moist condition: 125 lbs
-
Weight of soil after dry in oven: 100 lbs
-
Specific gravity of solid = 2.65
Requirements:
Determine void ratio, porosity, and degree of saturation
Problem solving technique:
- Void ratio, e = Vv/Vs (Vv, Vs, not given)
- Find Vs = Ws/gs (Ws = 100 lbs, gs is not given)
- Find gs = Gsgw (Gs is given, gw =62.4 lbs/ft3 is a know value)
- Find Vv = 1-Vs (e can be calculated)
- Porosity, n = Vv/Vt (Vv from step 4, Vs from step 2)
- Degree of saturation, S = Vw/Vv (Vv from step 4, need to find Vw)
- Vw =Ww/gw (Ww, not given, gw=62.4 lbs/ft3)
- Find Ww = Wt – Ws (Both Wt, Ww are given)
Solution:
-
Solid unit weight, gs = Gsgw=2.65*62.4=165.4 lbs/ft3
-
Volume of solid, Vs = Ws/gs = 100/165.4=0.6 ft3
-
Volume of void = Vt – Vs = 1 –0.6=0.4 ft3
-
Void ratio, e = Vv/Vs = 0.4/0.6=0.66
-
Porosity, n = Vv/Vt = 0.4/1 = 0.4
-
Weight of water = 125-100=25 lbs
-
Volume of water, Vw = Ww/gw = 25/62.4=0.4 ft3
-
Degree of saturation, S = Vw/Vv = 0.4/0.4x100% = 100%.
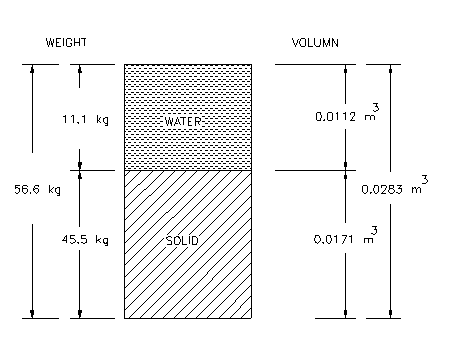
Example 4: Determine void ratio, porosity, and degree of saturation based on known volume, weight, and specific gravity (English units)
Given: (metric units)
-
Volume of soil mass: 0.0283 m3.
-
Weight of soil mass at moist condition: 56.6 kg
-
Weight of soil after dry in oven: 45.5 kg
-
Specific gravity of solid = 2.65
Requirements:
Determine void ratio, porosity, and degree of saturation
Problem solving technique:
- Void ratio, e = Vv/Vs (Vv, Vs, not given)
- Find Vs = Ws/gs (Ws = 45.5 kg, gs is not given)
- Find gs = Gsgw (Gs is given, gw =1 g/cm3 is a know value)
- Find Vv = 1-Vs (e can be calculated)
- Porosity, n = Vv/Vt (Vv from step 4, Vs from step 2)
- Degree of saturation, S = Vw/Vv (Vv from step 4, need to find Vw)
- Vw =Ww/gw (Ww, not given, gw=62.4 lbs/ft3)
- Find Ww = Wt – Ws (Wt = 56.6 kg, Ws = 45.5 kg are given)
Solution:
-
Solid unit weight, gs = Gsgw=2.65*1=2.65 g/cm3 = 2650 kg/m3
-
Volume of solid, Vs = Ws/gs = 45.5/2650=0.0171 m3
-
Volume of void = Vt – Vs = 0.0283 –0.0171=0.0112 m3
-
Void ratio, e = Vv/Vs = 0.0112/0.0171=0.65
-
Porosity, n = Vv/Vt = 0.0111/0.0283 = 0.39
-
Weight of water = 56.6-45.5=11.1 kg
-
Volume of water, Vw = Ww/gw = 11.1 kg/1 g/cm3= 11100 cm3= 0.0111m3
-
Degree of saturation, S = Vw/Vv = 0.0111/0.0111x100% = 100%.
 Download Files
Download Files
 Read also
Read also
- Solution of Soil Compaction Check Via The Voids Ratio
- Solution of Unit Weight and Its Conversion from Metric Units to SI and US Units.
- Solution of The Value of The Moisture When Fully Saturated
- Construction phases of Bituminous/Asphalt roads
- 7 Types of roads on the basis of material used
 Share
Share
Was this page helpful?
![]()
![]()
Follow our official Facebook page (@civilengineeringbible) and Twitter page (@CivilEngBible) and do not miss the best civil engineering tools and articles!
Posted by: ashaashamendocae0271155.blogspot.com
Source: https://civilengineeringbible.com/subtopics.php?i=6
Post a Comment for "Three Phase Diagram Geotechnical Engineering"